The polar curves \(r=1-\cos{\theta}\) and \(r=1+\cos{\theta}\) are shown in the figure above. Which of the following expressions gives the total area of the shaded regions?

Summary Submit

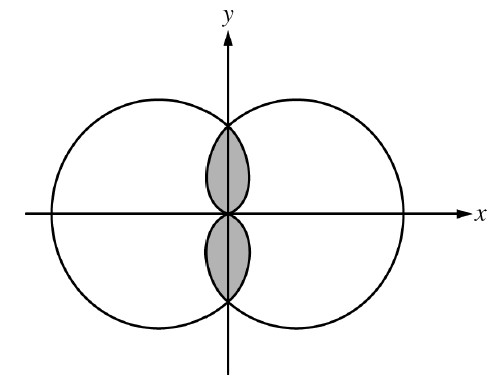
The formula for area bounded by the graph is:

$$ \frac{1}{2}\int \limits_a^b (r(\theta))^2   d\theta $$

If we can obtain the area for one of the four quadrants, we can simply quadruple that value.

The following integral provides the area for the top right quadrant of the cardioid (\(r=1-\cos{\theta}\)):

$$ \frac{1}{2}\int\limits_{0}^{\pi/2} (1-\cos{\theta})^2   d\theta $$

Quadrupling this value results in the answer:

$$ 4\cdot \frac{1}{2}\int\limits_{0}^{\pi/2} (1-\cos{\theta})^2   d\theta = \boxed{2\int\limits_{0}^{\pi/2} (1-\cos{\theta})^2   d\theta}$$

The other answer choices represent other areas (like the larger regions) or do not have the right coefficient to reflect additional regions.