In the figure shown below, square is inscribed in square . The length of is inches, and the length of is inches. In terms of and , which of the following expressions gives the area, in square inches, of ?
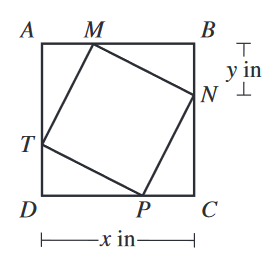
In the figure shown below, square is inscribed in square . The length of is inches, and the length of is inches. In terms of and , which of the following expressions gives the area, in square inches, of ?
