In the standard coordinate plane below, a shaded square is shown with vertices at , , , and . Two lines, and , each intersect the shaded square at exactly 1 point. Given that , what is the positive difference of and ?
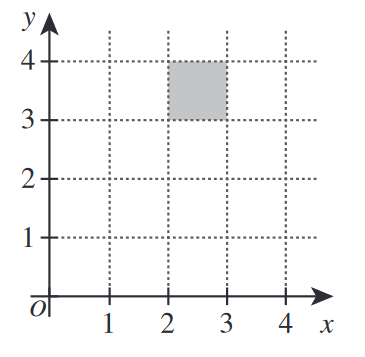
In the standard coordinate plane below, a shaded square is shown with vertices at , , , and . Two lines, and , each intersect the shaded square at exactly 1 point. Given that , what is the positive difference of and ?
