In the standard coordinate plane below, is bounded by , , and the -axis. Which of the following values is closest to the area, in square coordinate units, of ?
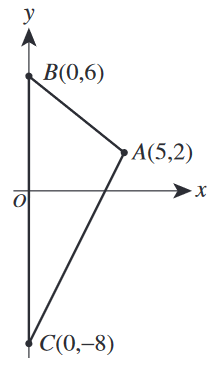
In the standard coordinate plane below, is bounded by , , and the -axis. Which of the following values is closest to the area, in square coordinate units, of ?
